An object is chiral if it differs from its mirror image. The favourite example is a hand: our right hands are reflections of our left ones. The two hands cannot be superimposed. The term chiral comes from 
According to Wikipedia, it was William Thomson, aka Lord Kelvin, who wrote:
“I call any geometrical figure, or group of points, ‘chiral‘, and say that it has chirality if its image in a plane mirror … cannot be brought to coincide with itself.”
Chirality is important in many sciences, most notably in chemistry. In mathematics, chirality is the property of a curve, surface or solid such that it is not identical to its mirror image. The term is found in topology and, in particular, in knot theory.
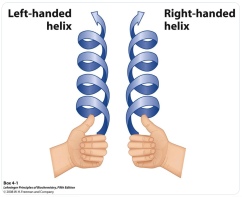
A helix may be left-handed or right-handed (see Figure). The DNA molecule is formed of right-handed helices, although it is not uncommon to see diagrams that use the left-handed versions. There are also left-handed and right-handed versions of the Möbius band.
Chiral and Achiral Knots
A knot is achiral if it can be continuously deformed into its mirror image. The unknot and the figure-of-eight knot are achiral. The unknot is essentially a continuously distorted circle (technically, via an ambient isotopy).
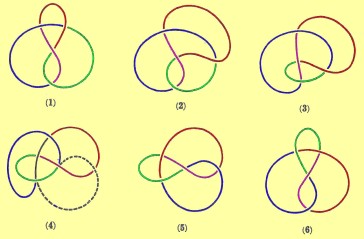
The Figure above illustrates how a figure-of-eight knot can be continuously transformed into its mirror image, demonstrating that it is achiral.
The trefoil knot is chiral: there are left-handed and right-handed trefoils (see Figure below) and it is impossible to transform one into another by a continuous deformation.
Imagine a bug confined to move within a thin flexible pipe closed by joining its ends. Assume that the pipe is in deep space. The bug can measure speed and acceleration; this is its only source of data.
Can the bug deduce the shape of the pipe and determine whether or not it is knotted? Comments to thatsmaths@gmail.com (Subject: Bug in pipe), preferably before the end of November 2021.
=================================================
That’s Maths II: A Ton of Wonders
by Peter Lynch
BARGIN: 40% OFF FOR NOVEMBER ONLY
€11.68 IF ORDERED DIRECT FROM LULU PRESS
GREAT CHRISTMAS GIFT!
>> Review in The Irish Times <<
=================================================
ThatsMaths 